Mark E. Curtis: contact
I would like to suggest a modification to the structure for the salt of deoxyribose nucleic acid (D.N.A.) that was proposed by Francis Crick and James Watson in April 1953.
In 1995 I began an investigation into the structure of DNA with the intention of producing a series of drawings and paintings of the double helix. My interest stemmed from certain features of my work as an artist, specifically my inquiries into the nature and depiction of space. In the manner of renaissance perspectival artists such as Uccello, I embarked on scale drawings of the helical structure using the standard textbook dimensions that derive from x-ray diffraction data. In the course of this work, discrepancies emerged, and it became clear to me that the Crick and Watson structure does not conform to geometric principles. Indeed my attempts to translate their theory from two into three dimensions ran into considerable topological problems.
Since the article in nature was published, their proposal would appear to have been fully vindicated by almost all-available empirical evidence. However, I have since discovered that a growing minority do recognise flaws in Crick and Watsons conclusions, specifically in terms of its topology and thereby the ability of the structure to replicate itself. Although some research has even gone so far as to question the very existence of the double helical structure itself, my proposal retains the double helix as its fundamental basis.
Without compromising the essence of their structure, I propose a resolution of the geometrical inconsistencies by means of a simple change in the position of alignment between the purines and pyrimidines. This realignment is founded entirely upon geometric principles and further investigation revealed a series of mathematical equations that describe a three-dimensional geometric helical space that conforms to the known ratios of DNA. In this paper, I will demonstrate the mathematical basis for this re-reading of DNA and moreover the simplicity and purity it engenders, when applied to the molecular structure of the base-pairing.
The Double Helix
The structure of the salt of deoxyribose nucleic acid is undoubtedly a double helix, with ten bases to each turn and an individual rotation of 36 degrees. Moreover, the approximate dimensions of a complete turn of DNA’s helix are well known: the diameter of the helix is 20Å (angstroms), the base height is 3.4Å and thus the helix extension is 34Å. These data enabled a simplified and systematic perspective projection of the structure, see Fig 1 (below).
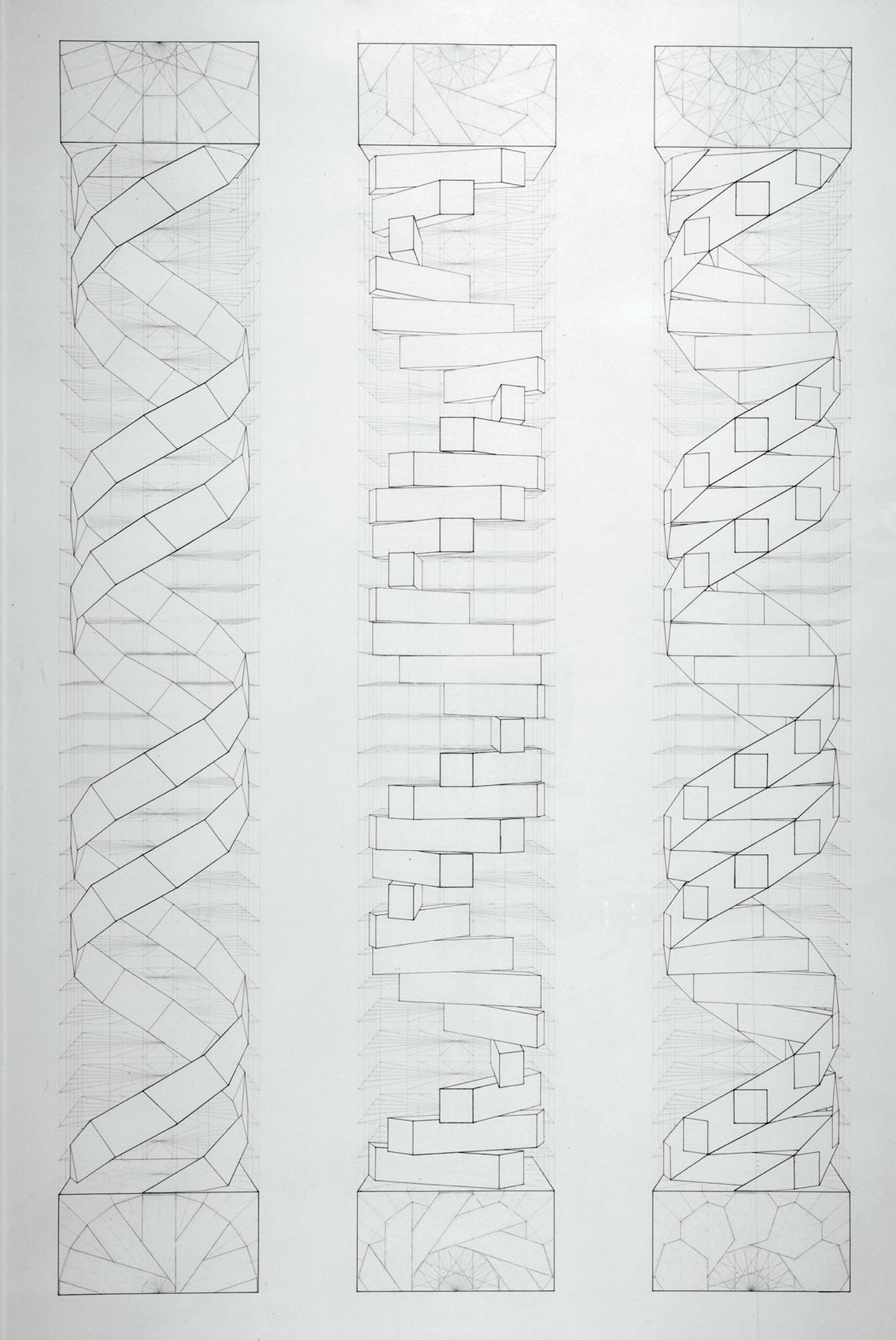
Fig 1
The Mathematics
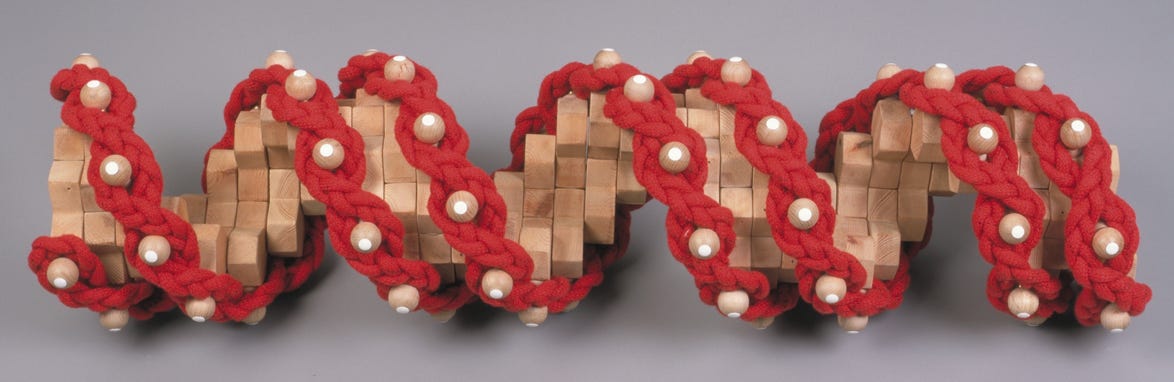
Although, theoretically, it is possible to construct helices from almost any series of polygons, there happens to be only one polygonal formation that would fulfil all the necessary criteria: ten regular pentagons orientated about a decagon, see Fig 2 (below).
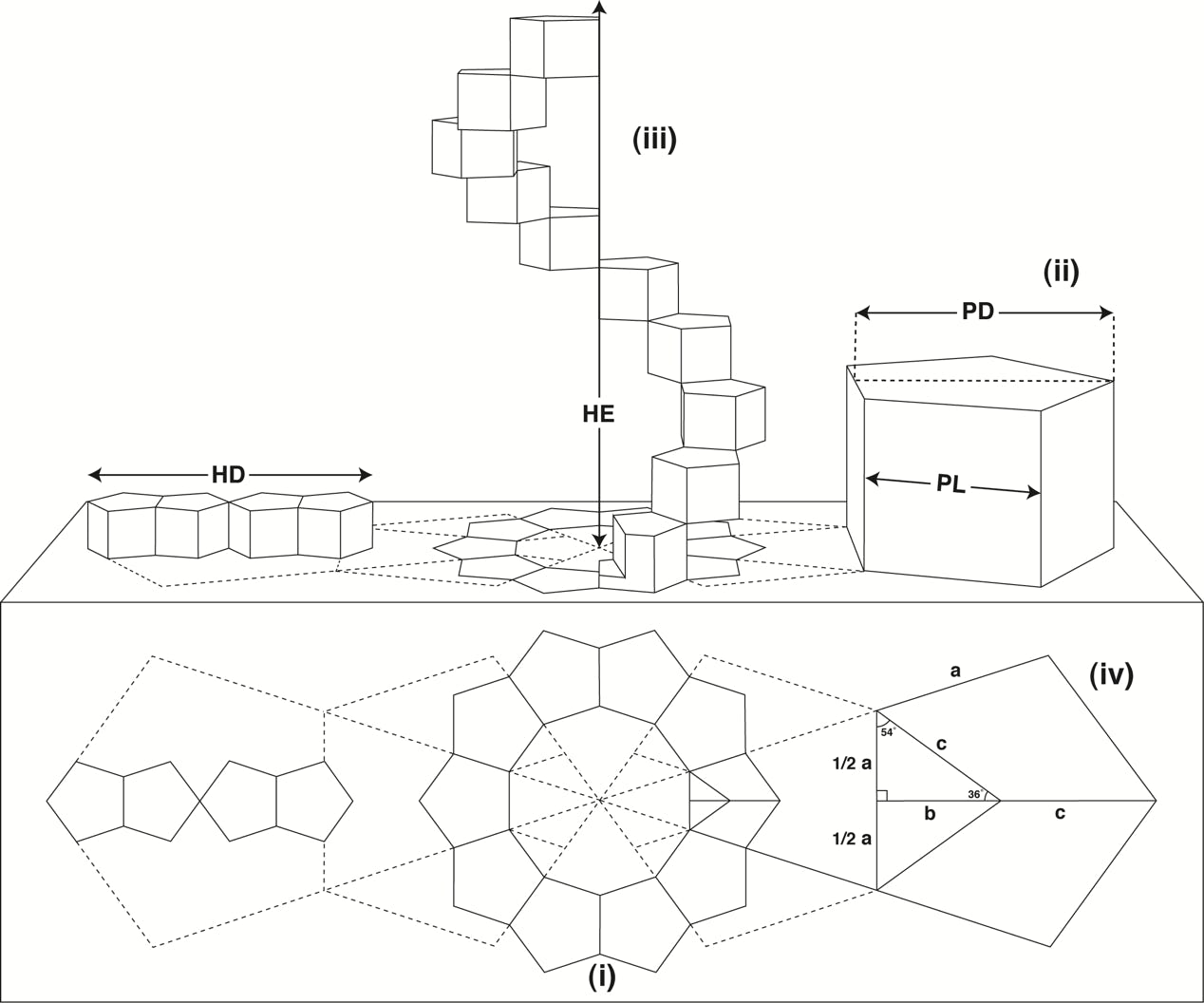
Fig 2
When translated into three-dimensional space, the pentagons become prisms with all lengths equal (ii).
A complete turn of the helix is formed by progressive rotation and extension of the ten regular prismatic pentagons (iii).
The diameter of the helix (HD) and the height of the helix extension (HE) have a direct and constant proportional ratio to the pentagon length (PL) and pentagon diameter (PD).
In order to establish the precise nature of this ratio we must devise some trigonometrical equations.
The values of a b c (iv) are ascertained as follows:
1/2a = c × sin 36°
b = c × cos 36°
c = 1/2a ÷ sin 36° or b ÷ cos 36°
The values of PL and PD may be determined as follows:
PL = 2 × 1/2a = a
PD = b + c
It follows, therefore, that:
HD = 4 × (b + c)
HE = 10 × a
The above equations enable us to establish the mathematical constant ratios correct to ten decimal places:
PL = 1
PD = 1.5388417686
HD = 6.1553670744
HE = 10
From these ratios, it is possible to come by a set of interrelated equations that would determine any of the measurements of a helix constructed from regular prismatic pentagons:
PL = PD ÷ 1.5388417686 or HD ÷ 6.1553670744 or HE ÷ 10
PD = PL × 1.5388417686 or HD ÷ 4
HD = PL × 6.1553670744 or PD × 4
HE = PL × 10
We are now in a position to apply any one of the known dimensions of DNA to the above equations. For example, if the PL – i.e. the base height – is known to be approximately 3.4 Å:
PD = 3.4 (PL) x 1.5388417686 = 5.23206201324 Å
HD = 3.4 (PL) x 6.1553670744 = 20.928248053 Å
HE = 3.4 (PL) x 10 = 34Å
These figures would appear to cohere with the known dimensions of DNA as revealed by the x-ray diffraction data and we now find ourselves in a position to construct an exact dimensional composite of the DNA double helix. In fig 2(above) I used single prismatic pentagons to illustrate the 3-dimensional helix. However, to ensure stability in the structure as it would exist in reality, it is necessary to place adjacent pentagons on each plane and then stack accordingly, thereby creating the ‘double helix’. Fig 3 (below).
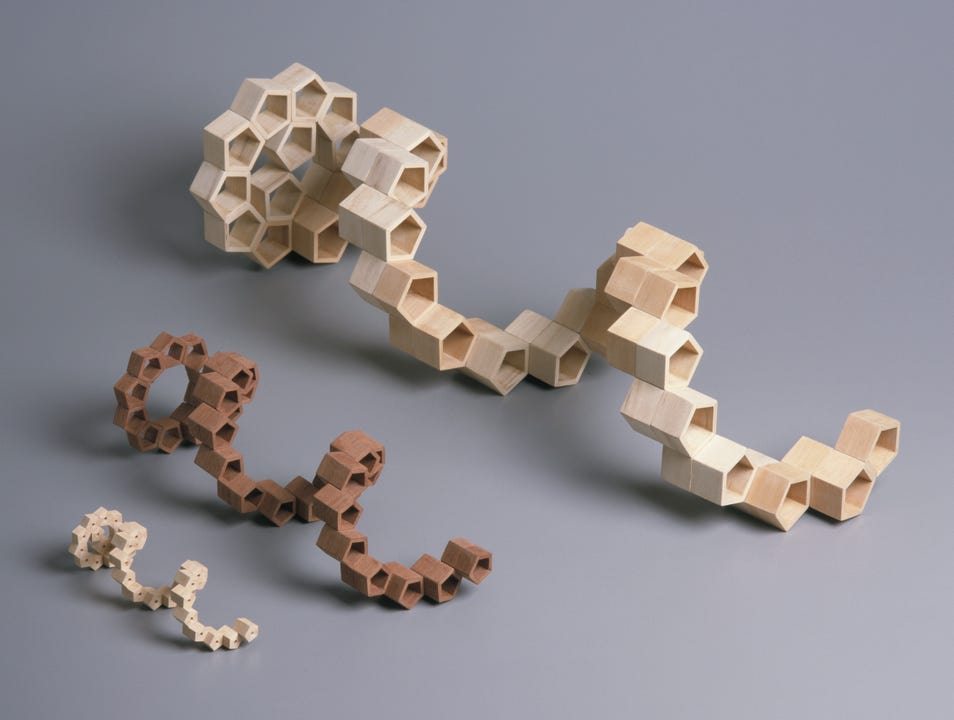

Fig 3
The application of geometry to the DNA molecular structure
The pairing of purines and pyrimidines in the Crick and Watson proposal locates the molecular pentagons that make up a part of the purines on the outside of the paired bases Fig 4 (below). However, it has been demonstrated that a more fluent and natural geometry would require ‘adjacent pentagons’ at the heart of the base pairing. It is therefore necessary to re-orientate the established molecular pairing, so that the pentagons of adenine (A) and guanine (G) form hydrogen bonds to the hexagonal structures of both thymine (T) and cytosine (C) Fig 5 (below).
This spatial arrangement of the hydrogen bonds enables the necessary adjacent second pentagon to become viable. Moreover, it also accords with our understanding of molecular bonding and maintains Chargaff’s base pairing ratios - the specificity of G with C and A with T.
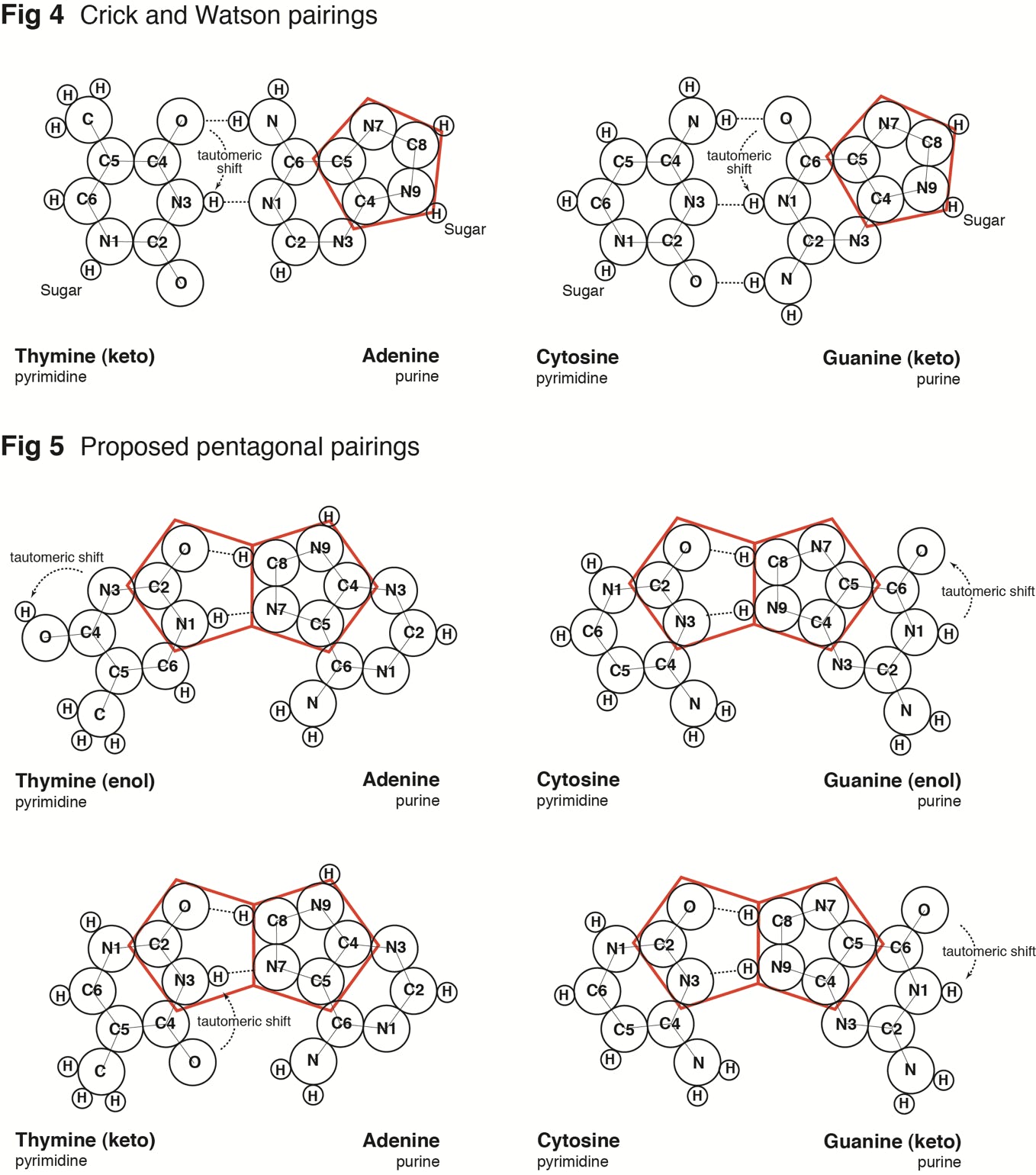
Fig 5
It would seem appropriate, at this point, to refer to the different formations that the molecules of guanine and thymine can take, namely the enol and keto states. Guanine and thymine may exist in either enol or keto configurations and are believed to pass through tautomeric shifts from one to the other – in other words, an indiscernible and spontaneous leap by the hydrogen atom from a specific oxygen atom to a specific nitrogen atom or vice-versa. The Crick and Watson proposal requires the keto formation, whereas this alternate proposal is viable in either formation.
In addition, it should be noted that presently the sugar-phosphate chains are depicted attached to N(1) of the pyrimidines and N(9) of the purines by covalent (glycosidic) bonds. However, both prior to 1953 and even today, when the base molecules are represented in isolation, hydrogen atoms are illustrated attached to these nitrogen atoms. For the purposes of this proposal, the four hydrogens are retained and one of them – guanine N(9) – now plays a practical role in the pairing of guanine with cytosine Fig 5 (above).
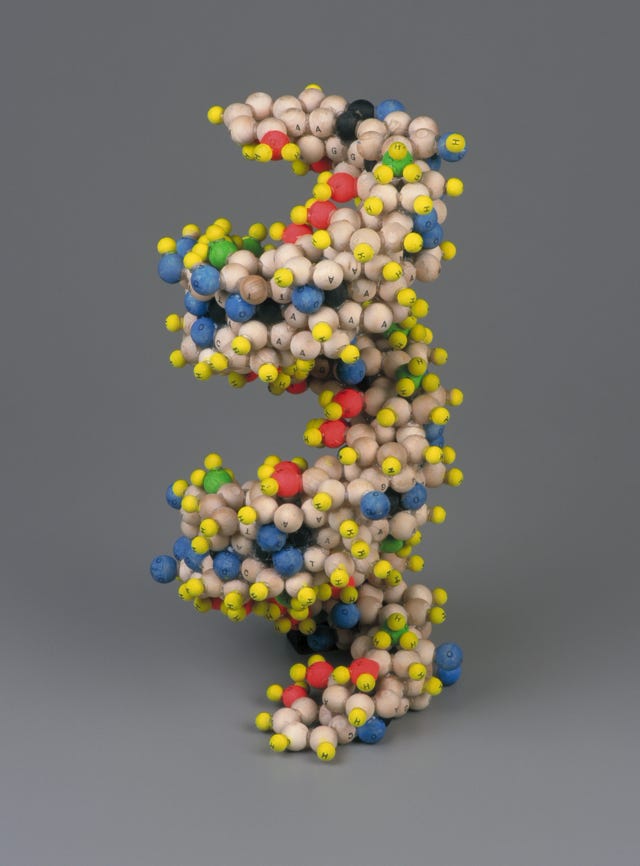
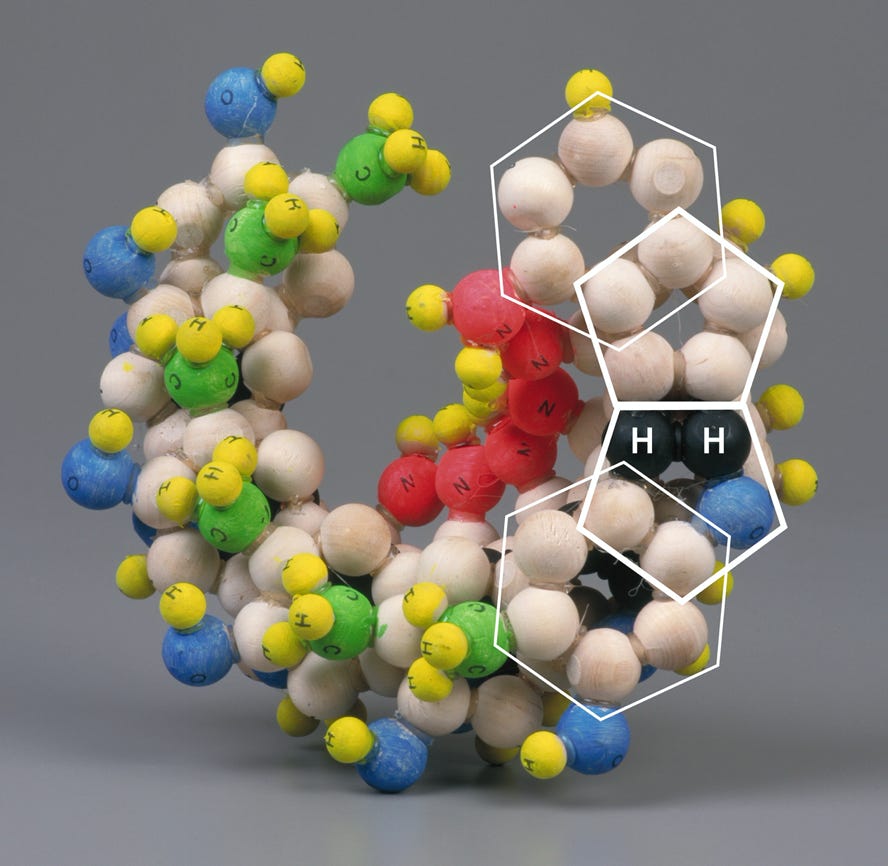
This alternative geometrical formulation reveals each base pairing to be an almost mirror image of the other, allowing a structure which, when fully assembled, exhibits both uniformity and stability. Most importantly perhaps, the alignment of the ‘spurs’ – that is to say, the NH2’s (G, C & A) or CH3 (T) – on each base pair is consistent. If either base pair were inverted, the spurs would naturally project in the opposite direction without upsetting the generative components of the helix. This ensures that the spurs attached to cytosine and adenine construct a constant, sequence specific, ‘secondary helix’ within the space generated by the primary helix; and that the spurs attached to guanine and thymine provide a sequence specific ‘capping’ of the ‘cavities’ generated by the conformation of the primary helix. These features not only display clear, predictable and simple mechanisms that may be utilised in the coding and articulation of amino acids but also a predictable braille like readability with which enzymes may be able to engage.



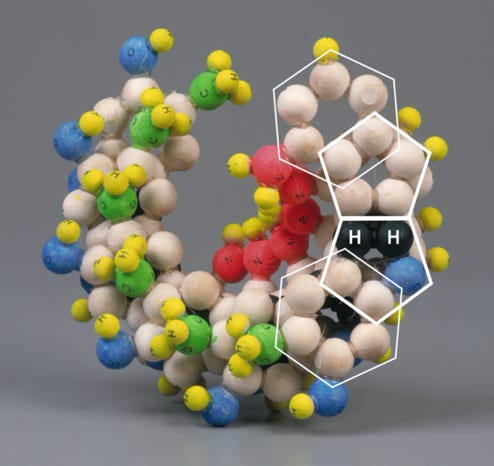
With respect to the sugar-phosphate chains, while it would be possible to incorporate covalent bonds into this proposal, to my mind, the nature of this revised structure does suggest a strong possibility that the sugar phosphate chains may be secured by means of multiple ‘hydrogen bonds’. The sheer quantities of accessible hydrogen and oxygen, both on the outside of this revised structure and also on the sugar-phosphate chains themselves, would potentially tie in with such a suggestion. Inevitably the first point of separation would continue to be the two hydrogen bonds that hold purine to pyrimidine, as those bonds would have considerably less resistance than the multiplicity of bonds that would hold the base-pair to the sugars and phosphates.



Conclusions
This proposed structure for DNA is wholly founded upon mathematical principles. Although the geometrical modification to the base pairings is relatively minor, the resulting double helix manifests a clarity altogether distinct from that offered by Crick and Watson and would appear to shed light upon a number of areas of continuing uncertainty.
The implications of these findings are inevitably far-reaching and would potentially affect many areas of research. However, these lie beyond my capacity to evaluate and I will restrict myself to reiterating the principal features of this proposal:
• Geometric equations predict the dimensions of DNA’s structure. Not only does the pentagonal geometry anticipate the helical dimensions but it also demonstrates a principle causation.
• The pentagonal geometry provides the architectural dynamics required to build a consistent, stable and uniform right or left handed helical structure and establishes why there should be consistently ten bases contained within a single turn of the helix. Incidentally, when converted to the molecular dimension I would certainly predict degrees of variation, certainly between 9.5 and 10.5 bases per turn, but perhaps even more.
• Both the hollow centre and side-by-side structural formation ensure instant access at any point within the helix. This would permit the DNA (even circular) to open and close during its replication functions without entangling itself.
• This modification to the base pairing would appear to be able to exist in either the enol or keto formation.
• While the sugar-phosphate backbones will undoubtedly prove integral to both the stability and perhaps the right handed orientation of the helical structure, it is the geometry of the base-pair molecules themselves that are ultimately responsible for the formation of the helix.
I have now set down a reinterpretation of DNA’s structure as I see it. It should be remembered that, by necessity, I publish this paper in my capacity as an artist and in the knowledge that nothing I have set down has yet been subjected to scientific scrutiny. It should be read as such, allowing the visual material to complement the text and vice-versa. This, after all, was the trigger for my initial interest. Aware of the further implications, I offer it in the hope that it may stimulate wider research and debate.
Mark E. Curtis 1997
Papers
Crick, F. & Watson, J. D. (1953) Nature, vol 171, 737-738. http://www.nature.com/nature/journal/v171/n4356/abs/171737a0.html
Gamow, G. (1954) Nature, vol 173, 318.(13 February 1954); http://www.nature.com/nature/journal/v173/n4398/abs/173318a0.html
Furberg, S. (1949) Nature, vol 164, 22. http://www.nature.com/nature/journal/v164/n4157/pdf/164022a0.pdf
Pauling, L. & Corey, R. B. (1953) Proc. Natl. Acad. Sci, 39, 84-96 http://www.ncbi.nlm.nih.gov/pmc/articles/PMC1063734/
Hoogsteen, K. (1959) Acta. Cryst.vol12, 822-823 doi:10.1107/S0365110X59002389
Hoogsteen, K. (1963) Acta. Cryst.vol16, 907-916 doi:10.1107/S0365110X63002437
Root-Bernstein, R. (1996) ‘Do we have the structure of DNA right’ Art Journal, SP 47-55.
Bansal, M. (2003) Current Science, vol 85, 1556-1563.
Books
Levene, P. A. & Bass, L. W. (1931) ‘Nucleic Acids’.
Todd, A. (1956) ‘Nucleic Acids’.
Thompson, D’arcy (1961) ‘On Growth and Form’ abridged edition.
Watson, J. D. (1968) ‘The Double Helix’.
Olby, R. (1974) ‘The Path to the Double Helix’.
Freeland Judson, H. (1979) ‘The Eighth Day of Creation’.
Crick, F. H. C. (1989) ‘What Mad Pursuit’.
Maddox, B. (2002) ‘Rosalind Franklin: The Dark Lady of DNA’.
Feyerabend, P (1975) ‘Against Method’
Websites
www.dna.place - snakes & ladders: musings on DNA structure by Sean Kettle
http://wellcomelibrary.org/using-the-library/subject-guides/genetics/makers-of-modern-genetics/
http://en.wikipedia.org/wiki/Hoogsteen_base_pair
http://en.wikipedia.org/wiki/George_Gamow
Addendum
Since my first offering of this proposal in 1997, it has met, perhaps not altogether surprisingly, with a decidedly cool response. There appears not only to be indifference but also a degree of outrage at my presumption. I should like to stress that I have presumed nothing and that I have been scrupulous in restricting this paper to that which geometry, chemistry and rational thought would defend. In this regard alone I am no less convinced of the case for more research to be undertaken. I therefore continue to maintain what I have done so already, with complete confidence, and would offer the following quote from Plato as some justification for my apparent dogmatism.
“We must in my opinion begin by distinguishing between that which always is and never becomes from that which is always becoming but never is. The one is apprehensible by intelligence with the aid of reasoning, being eternally the same, the other is the object of opinion and irrational sensation, coming to be and ceasing to be, but never fully real. In addition, everything that becomes or changes must do so owing to some cause; for nothing can come to be without a cause. Whenever, therefore, the maker of anything keeps his eye on the eternally unchanging and uses it as his pattern for the form and function of his product the result must be good; whenever he looks to something that has come to be and uses a model that has come to be, the result is not good.”
Plato - Timaeus. (28)
I hope this site will stimulate further areas of research amongst those people with a more specialist knowledge and enquiry. In response to those whose minds appear fixed within the present paradigm and who would use its ‘issue’ to pick holes in some of the detail of this alternate proposal I can but quote Thomas Kuhn:
“ ...the choice between competing paradigms regularly raises questions that cannot be resolved by the criteria of normal science. To the extent, as significant as it is incomplete, that two scientific schools disagree about what is a problem and what a solution, they will inevitably talk through each other when debating the relative merits of their respective paradigms. In the partially circular arguments that regularly result, each paradigm will be shown to satisfy more or less the criteria that it dictates for itself and to fall short of a few of those dictated by its opponent... The normal scientific tradition that emerges from a scientific revolution is not only incompatible but often actually incommensurable with that which has gone before... Because it has that character, the choice is not and cannot be determined merely by the evaluative procedures characteristic of normal science, for these depend in part upon a particular paradigm, and that paradigm is at issue.”
Thomas Kuhn - The Structure of Scientific Revolutions. Ch IX & XII
Science’s continuing dogmatic and almost deified belief in Crick and Watson will never be a substitute for truth and knowledge, and hence, within light of this geometry, I would suggest the re-evaluation of numerous papers, articles and books. Continuing use of the ‘Crick and Watson’ model as a ‘benchmark helix’ - with its faulty architecture and mechanics and its inaccurate atomic positioning appears tantamount to Nasa attempting an exploration of our solar system whilst believing in the geocentric system, epicycles and all. No true sense will be made either of genes or genomes until this geometry is addressed - there is simply far too much crucial information missing from their model. That the biochemist struggles to recognise and also to comprehend the validity of this work is perhaps symptomatic of their learned, and to all intensive purposes legitimate, belief in Crick and Watson’s opinion based model over so very many years - the possession of power and wealth invariably debases the free judgement of reason. With both their self-interest and their reason now fully developed and no longer free from the ‘unprejudiced senses’ and ‘preconceived notions’ that have evolved since 1953, it may well be that further exploration of this approach would be better suited to the pure chemist, biologist, physicist and mathematician. Engineers, architects, philosophers, designers, artists, theologians and nano-technologists could all potentially benefit from contemplation of this geometry in their respective disciplines. Indeed, everyone on planet earth ought to contemplate this structure - be it only in order to gain the wherewithal to be able to hold some of these modern-day theories to account. From my own perspective it is evident that many of the problems inherent in the world today stem not from how we teach but what we are teaching - the world has gone wrong and will continue to do so long as our truth and knowledge remain in a state of corruption. I would argue that all secondary school children ought to be acquainted with Euclid and this geometry prior to their introduction to Science.
Lastly, I would like to emphasise that everything you see set down on this website could, and therefore would, have been on the table in 1953 alongside Crick and Watson’s model had Euclid’s Elements laid out this elegant geometry. Indeed, I genuinely don’t believe we would have found ourselves in such a situation had the ‘Ancient Greeks’ understood linear perspective or ‘Renaissance Artists’ done more work in solid geometry. Rosalind Franklin, Linus Pauling, Karst Hoogsteen and others who questioned the veracity of Crick and Watson’s hypothesis at the time would, I believe, have championed such a rigorous approach. Indeed Hoogsteen’s genuinely scientific and objective research between 1959 and 1963, now buried because it failed to back up the C/W hypothesis, supports just such a natural pentagonal geometry. It needs be reminded that their helical model came to be accepted not because of objective evidence but because no other proposal was forthcoming at the time. And on them winning the Nobel Prize some years later in 1962, no other scientist was going to question further, something that to all intensive purposes was done and dusted. Whilst the C/W model answers and confirms some basic points - that it is a double helix, operates antiparallel, fulfils Chargraff’s base pairing ratios etc - it falls a long way short of elucidating any working detail. Quite simply, as I say earlier, they can sequence and map as many ‘genes' and ‘genomes' as they like, but they will never make any true sense of them until they get the primary structure correct - and I’m afraid that to do that they will have to take a very large step back to where it all began.
To those who will inevitably question my ‘authority’, the use of quotation and its appeal to previous authority will, I hope, clearly illustrate why I feel it so important to get this geometry addressed.
I would also draw attention to the following contextual ‘quotes’.